Romain saw our post on our Tennis for Two project and decided he wanted to make his own. He ordered a preprogrammed microcontroller from us and got to work. Once he was done, he was kind enough to share build photos and circuit diagrams with us.
Since he was starting with a bare CRT rather than a full scope, he built a wood and plexiglass enclosure which shows off the electronics very nicely.
We’re glad to see really retro gaming getting the attention it deserves!
Ask any group of mad scientists how they plan to take over the world, and one of the answers that you just might hear is “With giant monsters!” And rightly so. Mucking with the workings of life itselfis one of the key skills in the mad scientist’s arsenal.
Of course, we need to start small. The easiest way to begin is by experimenting on plants. Not only is it much less expensive, but your laboratory will remain more pleasant to work at, since PETP protestors tend to be fewer in number than those from PETA.
Plant growth, in all of its different aspects, is regulated by a complex system of hormones (signal chemicals) that are responsible for phenomena as complex as geotropism and phototropism, rooting and branching, dwarfism, and seed dormancy. It turns out that many of these chemicals are actually used in horticulture and are relatively easy to obtain.
One of the most interesting chemicals to play with is Gibberellic acid, a well-known and easily available plant hormone that can be used to stimulate growth. It is available in liquid form from United Nuclear, and can induce frankly absurdgrowth in many types of plants.
In order to see results quickly, it’s nice to start with a plant that grows quickly. We chose to experiment with mung been sprouts, which ordinarily reach maturity (from a particular salad-eater’s perspective) in about five days.
Continue reading Mad Science 101: Inducing giantism in living organisms
Our bicycle frame lunch bag project is showing up in print in a couple of places. The August/September issue of Bust magazine is running the project along a couple of other bicycle-themed articles. It’s nice to see bicycle fashion spreads that don’t include spandex! I like the Schwinn they used for the article photo, too.
The bag is also included in a calendar for next year, Sewing: 2010 Day-to-Day Calendar, which just arrived in the mail today. There are two projects each week, and each project is printed on an 8 1/2″ x 11″ sheet of paper folded in half. The loose leaf sheets are stored in a magnetic closure box which converts to an easel, which also locks in place with a magnet. Magnetic boxes are awesome! The box would be great to reuse as a recipe box/stand, especially since you don’t have to use funny sized cards for it. The calendar format is a little odd, showing three or four days at a time, but that’s probably fine for a decorative calendar. The bike bag appropriately shows up on Earth day. I appreciated that the calendar included a project index, and that every project author was listed with their website so you can look up their other projects, too.
The original project post is here.
Related projects:
Here we show you how to get started with water slide decals with a very silly (and rather expensive) Lego business card for those of us who don’t have mini-fig business cards.
Some time ago we came across a subtle magnetic nail polish. It had fine magnetic dust in it, and could record the local magnetic field profile at the time that the polish dried.
But hey, why can’t you do this with full-on iron filings? So, for our own bold and impractical take on this concept, we tried mixing genuine iron filings with nail polish (clear, in this case). Mix well, paint on, hold finger over (large) magnet while it dries. Don’t even think about trying to fit those spiky fingertips into gloves.
Results? So-so. The particles aligned with the field and solidified, but have more clumping than we’d like to see.
Maybe slightly finer particles would have been better. Much better would be if we found a good way to work with ferrofluid that could be hardened, or perhaps a version of magnetic viewing film that could be painted onto surfaces. Or maybe, if our version above were redone with RTV silicone, the particles could wiggle around in the presence of an external field.
We leave these important questions to higher minds than our own.
It’s like one of those online “LED resistance calculator” thingies, except that you can fold it up and put it in your wallet.
Continue reading Wallet-size LED Resistance Calculator
We were recently contacted by a mathematics instructor, who suggested that it might be interesting to have a program like Snowflake, but with the option of picking and choosing different symmetry properties.
Natural snowflakes have (approximate) sixfold rotation symmetry plus reflection symmetry. However, a lot of things that you can draw by hand have absolutely no resemblance to snowflakes at all– and it is somewhat fun to explicitly play with the rules.
Our new program, SymmetriSketch, sticks to the same basic design principles as Snowflake: it’s cross platform, open source, and able to export a true vector drawing with a closed path. However, SymmetriSketch is a much more flexible program that allows you to play with different symmetries, and create all kinds of different things that would never be mistaken for frozen water.
Here’s what it looks like when the program first opens:
The initial shape is an overall pentagon– an object with five-fold rotation symmetry and reflection symmetry. The figure is generated by taking the editable slice– highlighted here and when you start the program– and reflecting and rotating it to complete the full shape that you see.
Within the editable slice, you can also see three highlighted control points that can be dragged around. There is control point at every vertex and at the midpoint of every line segment between two vertices. If you drag a control point that is the midpoint of a line segment, it turns that control point into a new vertex. That new vertex also gets new control points at the midpoints to its neighbors.
Every vertex point can be moved to any location on the screen with the exception of the vertex that is initially at the top point of the pentagon– that vertex is constrained to move along the vertical axis– the axis of reflection symmetry.
The controls are purposefully kept simple. There are two symmetry controls– for the order of rotational symmetry and to toggle reflection– which you can change in the lower left hand corner of the screen.
The number, with its +/- controls, refers to the order of discrete rotational symmetry. If the number shown is n, then n-fold rotational symmetry is applied, which means that the displayed object is unchanged when rotated by 360 degrees/n. In the screenshot above, 9-fold rotational symmetry is applied.
Orders from 1 to 99 are allowed– note that 1-fold rotational symmetry is “no symmetry at all” since it requires 360/1 = 360 degrees of rotation to return to the original shape.
The second control is for reflection symmetry, and toggles between “reflect” or “rot. only,” where it either does, or does not apply a mirror reflection across the vertical axis.
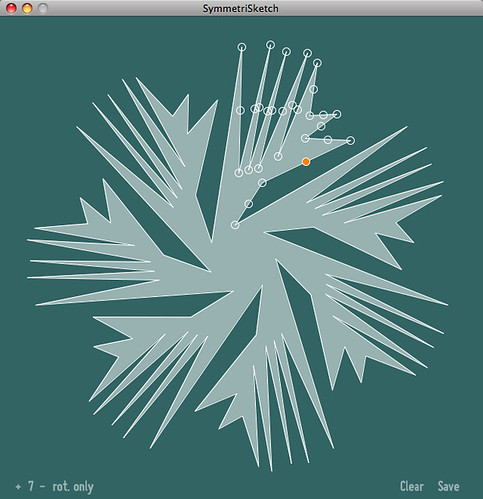
With reflection symmetry turned off, the figure is drawn with pure rotational symmetry. (This screenshot was taken while editing the shape, and you can see control points, indicated by little circles.)
Continue reading SymmetriSketch: A simple app for playing with symmetry
Photo by Brittany Turner
Brittany recently let us know that she had made skirt guards for her bike using my instructable. She used floral wire instead of cable ties since that’s what she had on hand. The guard following the shape of the rack is a nice touch, too.
We always love hearing about your projects and seeing your photos in the flickr auxiliary! Keep ’em coming!
